Want to test yourself against the most difficult SAT math questions given in the past 10 years? Want to know what makes these questions so difficult and how best to solve them? If you’re ready to really sink your teeth into the SAT math section and have your sights set on that perfect score, then this is the guide for you.
We’ve put together what we believe to be the 21 most difficult questions the SAT has given to students in the past 10 years, with strategies and answer explanations for each. These are all real, hard SAT math questions, which means understanding them is one of the best ways to study for those of you aiming for perfection.
Image: Sonia Sevilla/Wikimedia
Brief Overview of the SAT Math Section
The SAT math section is broken into three subsections, scattered throughout the test. Each math subsection is arranged in order of ascending difficulty, so on each subsection, question 1 will be “easy” and question 16 will be considered “difficult.”
The key except to this rule of progressive difficulty is in the math subsection that is a combination of multiple choice and grid-in answers. There, the multiple choice will be arranged in increasing difficulty (questions 1 and 2 being the easiest, questions 7 and 8 being the hardest) and then the difficulty level resets for the grid-in section (meaning questions 9 and 10 will again be “easy” and questions 17 and 18 will be very difficult).
The way the SAT classifies “difficulty” is by how long it takes an average student to solve a problem, as well as the percentage of students who answer the question correctly. As you might expect, the longer it takes to solve a problem and the fewer people who answer it correctly, the more difficult the problem.
So, with very few exceptions, the most difficult SAT math problems will be clustered in the far end of the multiple choice segments or the later half of the grid-in questions. Other than their placement on the test, these questions share a few other commonalities. Let us look at what these types of questions have in common, then look at example questions and how to solve them.
But First, Should YOU Be Focusing on the Hardest Math Questions Right Now?
If you’re just getting started in your study prep (or if you’ve simply skipped this first, crucial step), definitely stop and take a full practice test to gauge your current scoring level. Check out our guide to all the free SAT practice tests available online and then sit down to take a test all at once. The absolute best way to assess your current level is to simply take the SAT as if it were real, keeping strict timing and working straight through (we know--probably not your favorite way to spend a Saturday).
Once you’ve got a good idea of your current level and percentile ranking, you can set milestones and goals for your ultimate score. If you’re currently scoring in the 200-400 range, or the 400-600 range, your best best is to first check out our guides to improving your math score to where you want it to be before you start in trying to tackle the most difficult math problems on the test.
If, however, you are already scoring above a 600 and want to test your mettle for the real SAT, then definitely proceed to the rest of this guide. If you’re aiming for perfect (or close to), then you’ll need to know what the most difficult SAT math questions look like and how to solve them. And luckily, that’s exactly what we’re here for.
So is there anything the hardest questions have in common? The more you understand how they work, the easier they will be to solve whenever you come across them.
What Do the Hardest SAT Math Questions Have in Common?
Now, lastly, before we get to the questions themselves, it is important to understand what makes these hard questions “hard.” By doing so, you will be able to both understand and solve similar questions when you see them on test day, as well as have a better strategy for identifying and correcting your previous SAT math errors.
In this section, we will look at what these questions have in common and give examples for each type. In the next section, we will give you all 21 of the most difficult questions as well as answer explanations for each question, including the ones we use as examples here.
Some of the reasons why the hardest math questions are the hardest math questions are because the question:
- Tests several mathematical concepts at once.
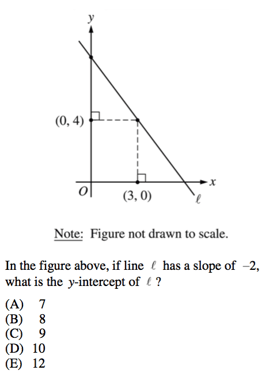
Here, we must deal with general principles of coordinate geometry, quadrilaterals, and slopes all at once.
- Tests one basic mathematical concept but makes you run through multiple steps to solve the problem. (Remember: the more steps you need to take, the easier to mess up somewhere along the line!)
We must solve this problem in steps to unlock the rest of the answers in a domino effect. This can get confusing, especially if you're stressed or running out of time.
- Uses concepts that you likely have limited familiarity with. For example, many students are less familiar with functions than they are with fractions and percentages, so most function questions are considered “high difficulty” problems.
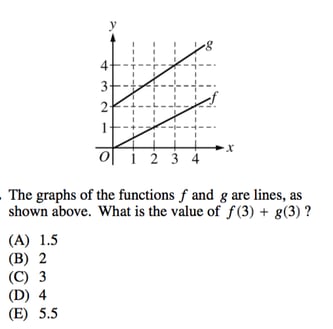If you don't know your way around functions, this would be a tricky problem.- Is worded in unusual or convoluted ways. It can be difficult to figure out exactly what some questions are asking, much less figure out how to solve them. This is especially true when the question is located at the end of the section and you are running out of time.
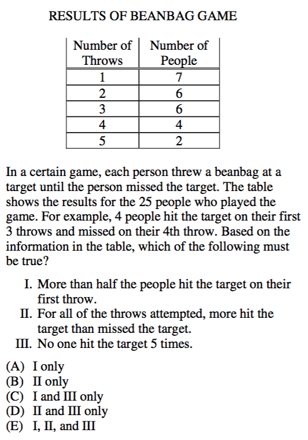
Because this is such an unusual and unclear question, it can be difficult to puzzle through in the limited time allowed.
- Gives a false appearance of being easy, tempting students to answer (resulting in a point penalty for an incorrect answer).
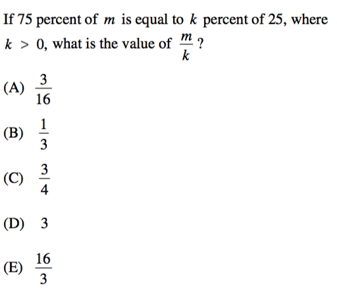
This question looks like a simple problem on percentages, but it is actually trickier than it appears. Many students fall for one of the bait answers provided and get the question wrong.
- Uses many different variables, both in the question and in the answer choices, or presents hypotheticals. (Note: the best way to solve these types of questions--questions that use multiple integers in both the problem and in the answer choices--is to use the strategy of plugging in numbers.)
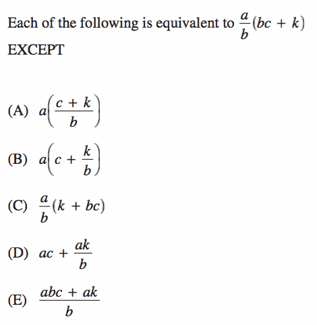
With so many different variables in play, it is quite easy to get one's equations muddled.
Now let's get to our list of questions (whoo)!
Image: Niytx/DeviantArt
The 21 Hardest SAT Math Questions
Now that we’ve seen some of the reasons why these questions are hard (and previewed some of the questions on the list), let’s dive right in!
1)
2)
3)
4)
5)
6)
7)
8)
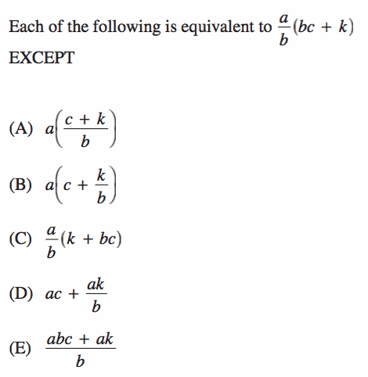
9)
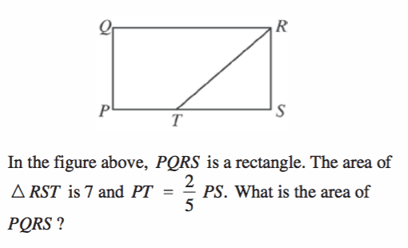
10)
11)
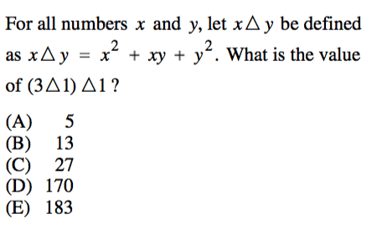
12)
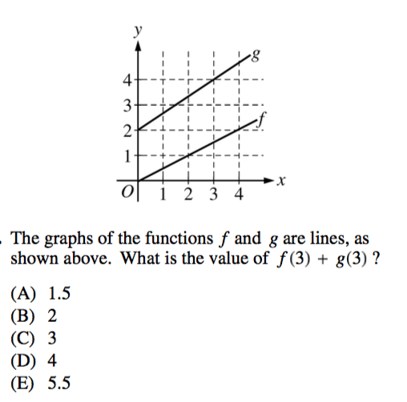
13)
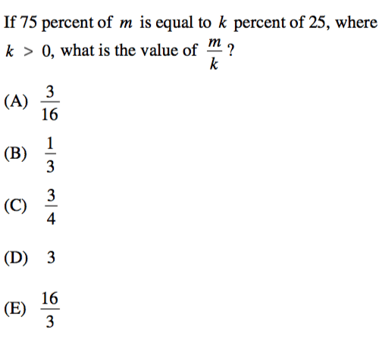
14)
15)
16)
17)
18)
19)
20)
21)
Want to improve your SAT score by 240 points? We've written a guide about the top 5 strategies you must be using to have a shot at improving your score. Download it for free now:
Answers: 1) B, 2) B, 3) C, 4) A, 5) 700, 6) E, 7) A, 8) A, 9) $70/3$ or 23.3, 10) D, 11) E, 12) E, 13) B, 14) C, 15) A, 16) B, 17) D, 18) C, 19) 10, 20) $49/5$ or 9.8, 21) E
Answer Explanations:
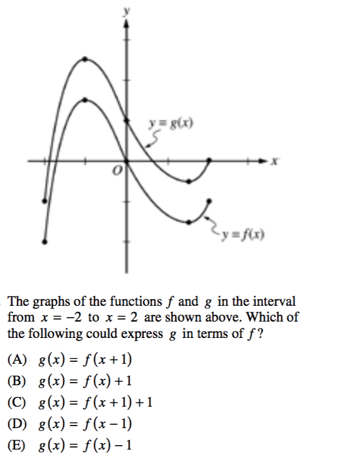
1) If we remember our rules of translations, we know that functions will move vertically upwards if we add to our output, and will move vertically downwards if we subtract from our output. This means we can eliminate answer choices A, C, and D, since they modify our input and the only difference between our graphs is a vertical shift (not a horizontal one).
Now we must express $g$ in terms of $f$, which means that we must add to the function $f$ in order to translate it into function $g$.
Our final answer is B, $g(x) = f(x) + 1$
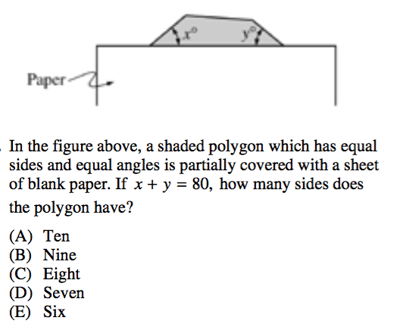
2) We know from our rules of quadrilaterals that any four-angled figure will have an interior degree sum of 360. If $x + y = 80$, the remaining angles will sum to be:
$360 - 80$
$280$.
Since we are told that the polygon is regular, each angle will be equal, which means that the individual angles of the larger polygon will be:
$280/2$
$140$
Now let's look at our answer choices. If you remember your polygon formulas, you know that the interior degree sum of a polygon will be $(n - 2)180$, with $n$ being the number of sides.
An eight sided figure will therefore have a interior sum of:
$6 * 180$
$1080$
Which means its individual angles will be:
$1080/8$
$135$
We need a figure with more sides, so let's try 9 next.
$7 * 180$
$1260$
And again, divide by the number of sides.
$1260/9$
$140$
Success!
Our final answer is B, our polygon has nine sides.
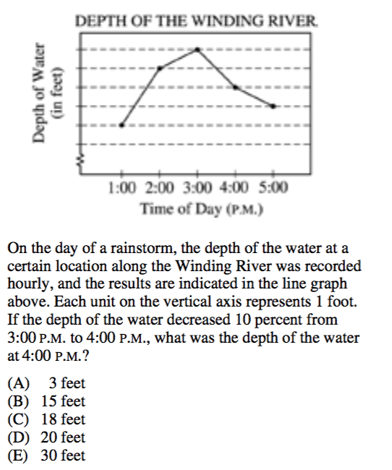
3) We are told that each vertical axis represents 1 foot and we can see that the water dropped 2 feet between 3 and 4 PM. We are told that this drop represents 10% of the original depth at 3 PM, so we must find what 2 feet is 10% of. This means we must set up a proportion.
$10/100 = 2/x$
$10x = 200$
$x = 20$
The water was 20 feet deep at 3 PM and dropped 2 feet by 4 PM. The water was therefore 18 feet deep at 4 PM.
Our final answer is C, 18 feet.
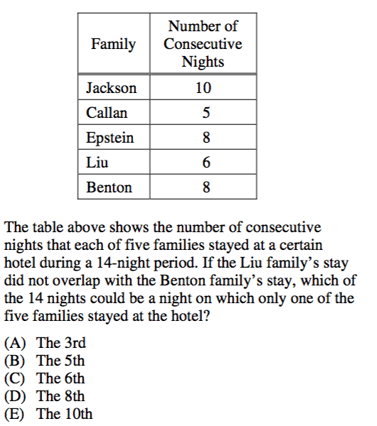
4) Because the Liu family's stay did NOT overlap with the Bentons', we know that either the Benton's stayed for the first 8 nights and the Liu family stayed the last 6 or the Liu family stayed the first 6 nights and the Benton family stayed the last 8. Because we are only working in a 14-day timeline, there are no other options. This means that there was NO night during which the hotel was unoccupied.
Now the family that poses the next most pressing obstacle is the Jackson family, as they stayed the longest. Because of their long stay, it will only be possible for ONE family (a family that does NOT overlap with the stay of the Jacksons) to have been at the hotel during the first four days or the last four days. This means that our only possible answer choices will be:
The 1st, the 2nd, the 3rd, the 4th, OR the 11th, the 12th, the 13th, or the 14th.
The only answer choice that matches any of those is answer choice A, the 3rd.
Our final answer is A, the 3rd.
5) Because Tom and Alison had the same number of sales and the same compensation during the week, this is actually a (complicated) single variable equation. So let us set up our equations and set them equal to one another.
Tom: $300 + 0.2x$
Alison: $200 + 0.25x$
$300 + 0.2x = 200 + 0.25x$
$100 = 0.05x$
$x = 2000$
Because $x$ represents their sales, they made 2000 dollars worth of sales for the week. But they do not get to keep all of this money, so now we must plug this figure back into one of the equations to find their actual weekly compensation. It doesn't matter which equation we choose--they will be equal.
$300 + 0.2(2000)$
$300 + 400$
$700$
Our final answer is 700, Tom and Alison each earned 700 dollars that week.
6) This question relies on our knowledge of our solid geometry rules and knowledge of how to properly use exponents. If our sphere has a radius of $r$, it must have a diameter of $2r$. Because the sides of the sphere touch the sides of the cube, this means that each side of the cube will also have lengths of $2r$.
We can find the volume of a cube by cubing the length of one of its sides, so the volume of our cube will be:
$(2r)^3$
$8r^3$
Our final answer is E, $8r^3$
7) We know that the only possible ways to get a product of 9 is for the last digits of our two numbers to be either:
$1 * 9$
OR
$3 * 3$
Because we are working with consecutive integers, it is impossible for $j$ and $n$ to both end in a units digit of 3. This means they must somehow end in a combination of 1 and 9.
The only way to have a combination of 1 and 9 in the units digits of consecutive integers a distance of two units apart is to have them come in the order of 9 and then 1. For instance, let us say that $j$ was 9. $j$, $k$, $n$ would therefore be:
$9, 10, 11$
Which means that the units digit of $k$ is 0.
Our final answer is A, 0.
8) Just by looking at our answer choices, we can see straight away that answer choices A and B are extremely similar, but NOT equivalent. This means that our final answer will either be A or B.
If we multiply $a/b$ by $b/c$, we will get:
${ab}/{bc}$
$ac$
We can see, then, that our $b$'s will cancel each other out. This means that we will NEVER have a fraction with $c/b$ in any of our equivalencies. Even without going any further, we can see that option A is incorrect.
[Note: an alternate way to answer this question is to use the strategy of plugging in your own numbers. That way you won't have to deal with multiple variables and can use real numbers instead. This can be invaluable for those of you who are uncomfortable using algebra or those of you who just want to double-check your answer.]
9) We know that we can find the area of a triangle using the formula $a = {1/2}bh$. We are also told that PT is $2/5$ths of PS, which means that TS is worth $3/5$ths of PS. (For simplicity's sake, we can just imagine that the full length of PS is 1.)
Because PS also works as the base of triangle, we can find the height using our triangle formula. So:
$7 = {1/2}(3/5)h$
$7 = {3/10}h$
$70/3 = h$
Because we already said that the full length of PS was 1 and we have just found the height of the rectangle (length RS), we can say that the area of the rectangle is:
$70/3 * 1$
[Note: even though saying PS has a length of 1 means that the picture is not to scale, this doesn't matter! For instance, we could have said that PS was 5, meaning that PT was 2 and TS was 3. If you work through the equation again using these numbers, you will still get the same final answer as we got here.]
Our final answer is $70/3$ or 23.3.
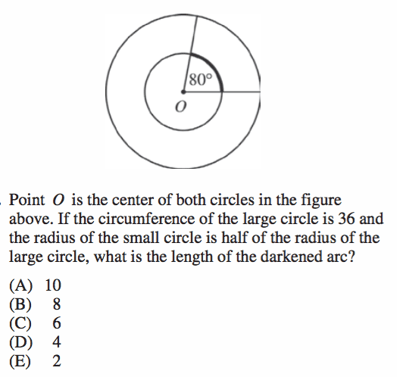
10) We know from our circle formulas that the circumference of a circle is $2πr$. This means that, if our larger circumference is 36, the larger radius must be 18.
The radius of the smaller circle is half the radius of the larger, which means it must be 9.
Now, we find the arc of a circle by multiplying the full measure of the circumference by the fraction of the circle that the arc spans. So:
$c_{arc} = 2πr(80/360)$
$18π(0.2222)$
$4π$
Our final answer is D, 4.
11) As with any algebra or function problem, we must first start inside out. We are given the definition of △, so let us start with the part of the expression in parenthesis.
$3△1$
$3^2 + (3)(1) + 1^2$
$9 + 3 + 1$
$13$
Now our expression is:
$13△1$
$13^2 + (13)(1) + 1^2$
$169 + 13 + 1$
$183$
Our final answer is E, 183.
12) Our two functions are essentially asking us, “What is the y-axis value when the x-value is at 3?”
So if we look at the y-coordinate value where each line has an x-coordinate value of 3, we can see that the y-axis value will be at 1.5 for function $f$ and at 4.0 for function $g$.
If we add these values together, we get $1.5 + 4 = 5.5$
Our final answer is E, 5.5.
13) We can either set this problem up using algebra or by plugging in our own numbers. For instance, if we chose a number for $m$ or $k$, we could find the value of the other and work with real numbers. In this explanation, however, we'll use algebra.
Let us start by setting up our percentage equation. We know that 75% of $m$ is equal to $k$ percent of 25, so if we write that out, we get:
$0.75m = 0.k25$
Because $k$ is a percentage, we must multiply it by 100 to get it to be worth $k$ amount (instead of $0.k$). To keep the equation equal, we must do the same for the 0.75 on the other side as well.
$75m = k25$
Now if we manipulate the equation to give us $m/k$, we will get:
${75m}/{k} = 25$
$m/k = 25/75$
$m/k = 1/3$
Our final answer is B, $1/3$
14) If our $x$ value is a positive integer (e.g., +2), answer choice A will be correct.
If our $x$ value is a negative decimal point (e.g., -0.5), answer choice B will be correct.
If our $x$ is a negative integer (e.g., -2), answer choice D will be correct.
If our $x$ value is a positive decimal point (e.g., +0.5), answer choice E will be correct.
There is no possible way for answer choice C to be correct.
Our final answer is C, $x^2 < x^3 < x$
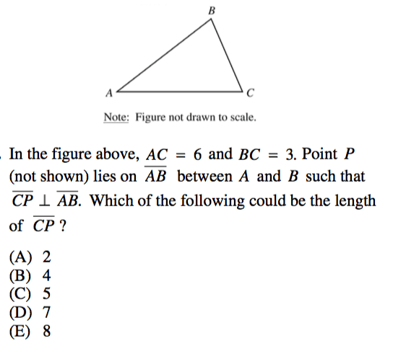
15) If we drew a picture of the line CP, we would form a right triangle CPB. Because BC = 3 and it is also the side opposite the 90 degree angle of triangle CPB, it must be the hypotenuse.
The hypotenuse will always be the longest side in a triangle, so leg lengths BP and CP would have to be smaller than 3.
The only answer choice that gives us a length of CP that is shorter than 3 is A, 2.
Our final answer is A, 2.
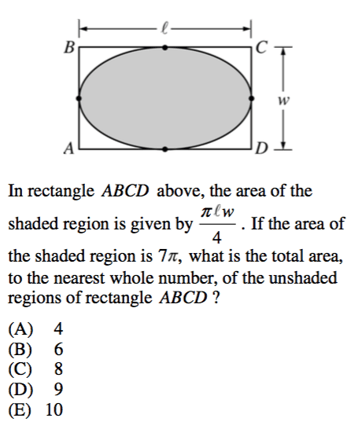
16) We are given our formula and the answer to that formula, so we can set them up to find our missing pieces.
$7π = {πlw}/4$
$28π = πlw$
$28 = lw$
Now $l * w$ is 28 and also the full area of the rectangle. This means we must subtract the area of the shaded region from the total area in order to find the combined area of the unshaded spaces alone.
$28 - 7π$
$28 - 21.99$
$≅6$
Our final answer is B, 6.
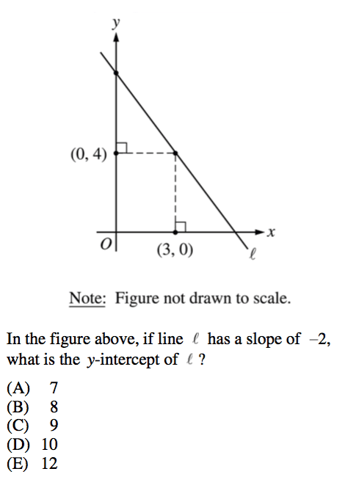
17) From our coordinates, we know that the height of the rectangle is 4 and the width of it is 3. This means that its upper rightmost coordinate point (the one touching line $l$) will be (3, 4).
From there, we can simply count upwards using the slope value of the line.
We know that a slope is ${rise}/{run}$ (in other words, the change in $y$ over the change in $x$), so we will need to continuously add 2 to our $y$-coordinate values and subtract 1 from our $x$-coordinate values as we travel leftwards. Once we hit $x = 0$, we will have found our point where $l$ hits the $y$-intercept.
1st point: (3, 4)
2nd point: (3 - 1, 4 + 2) => (2, 6)
3rd point: (2 - 1, 6 + 2) => (1, 8)
4th point: (1 - 1, 8 + 2) => (0, 10)
The $y$-intercept is 10.
Our final answer is D, 10.
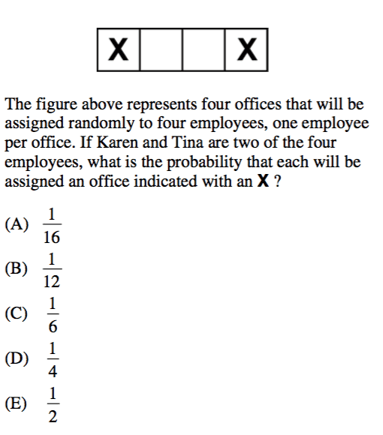
18) This is a probability question in which our options change as certain outcomes occur. This means we must be careful to keep track of what has already happened.
The odds of one of the women getting one of the $x$-marked desks will be $2/4$ or $1/2$.
The odds of the second woman being assigned to the remaining $x$-marked desk will now be $1/3$ (Why? Because one of the $x$-marked desks has already been taken by the first woman).
Now the odds of BOTH events occurring (a probability combination) will be the product of these two outcomes. So:
$1/2 * 1/3$
$1/6$
Our final answer is C, $1/6$.
19) The easiest way to visualize this problem is to simply replace $m$ with out own number. So let us find a number that has a remainder of 3 when it is divided by 5. In this case, we will say that $m = 8$ (Why 8? Any number with a units digit of either 3 or 8 will work, so why not!)
If $m = 8$, $20m$ will be 160.
$160/25$
$6r10$
Our remainder is 10.
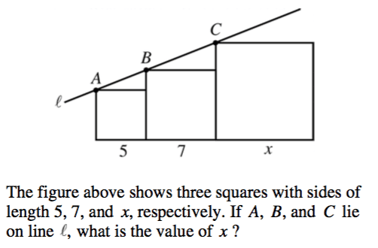
20) Because we are dealing with a straight line connecting a series of squares, the spaces in between the line and the boxes will form a series of right triangles. We can therefore set up a proportion to find the missing height value of the triangle for the third box.
The first square has a width of 5, which means it must also have a height of 5 (squares have equal lengths and widths). As it is standing exactly next to a square with a width (and therefore height) of 7, the height of the triangle between them must be 7 - 5 = 2.
Now we can set up a proportion between the squares and the heights of their connecting triangles to find the missing second triangle height.
$5/2 = 7/y$
$5y = 14$
$y = 2.8$
Now this is the height of the space between the second box and the third, which means that the total height of the third box will be:
$2.8 + 7$
$9.8$
21) This is a very unusual way to present a problem, so it can take a second to puzzle through (especially with adrenaline running through your veins!). We are told that 25 people played the game and the right column marks how many people missed on a certain throw.
This means that 7 people missed on their first throw and 18 people hit the target. 18 is more than half of 15, so option I is correct.
It also means that 7 people threw the bag one time only and missed each time. So far this gives us 7 misses (-7).
6 people threw it twice, meaning they hit it once and then missed on their second attempt. This gives us +6 for the hits and -6 for the misses.
Another 6 people threw it three times, meaning they hit it twice and then missed on their third attempt. This gives us +12 and -6.
4 people threw it four times, meaning they hit it three times and then missed on their fourth attempt. This gives us +12 and -4.
2 people threw it five times, meaning they hit it four times and then missed on their fifth attempt. This gives us +8 and -2.
If we add up all the misses and hits, we get:
$-7 + 6 - 6 + 12 - 6 +12 - 4 + 8 - 2$
$13$
There were more hits than misses, so option II is also correct.
Now, we don’t even NEED to verify option III, since the only way to have options I and II both be correct is with answer choice E. But we can quickly verify it regardless just by looking at the chart.
We already know that there were 25 participants and have also established that no one on the chart hit the target five times. If we count everyone in the “number of people” column, we can see that all 25 participants are accounted for, meaning that option III is indeed correct.
Our final answer is E, I, II, and III.
You deserve all the naps after running through those questions.
The Take-Aways
The SAT is a marathon and the better prepared you are for it, the better you'll feel on test day. Knowing how to handle the hardest questions the test can throw at you will make taking the real SAT seem a lot less daunting.
If you felt that these questions were easy, make sure not underestimate the effect of adrenaline and fatigue on your ability to solve problems. As you continue to study, always adhere to the proper timing guidelines and try to take full tests whenever possible. This is the best way to recreate the actual testing environment so that you can prepare for the real deal.
If you felt these questions were challenging, be sure to strengthen your math knowledge by checking out our individual math topic guides for the SAT. There, you'll see more detailed explanations of the topics in question as well as more detailed answer breakdowns.
What’s Next?
Felt that these questions were harder than you were expecting? Take a look at all the topics covered on the SAT math section and then note which sections you had particular difficulty in. Next, take a gander at our individual math guides to help you shore up any of those weak areas.
Running out of time on the SAT math section? Our guide will help you beat the clock and maximize your score.
Aiming for a perfect score? Check out our guide on how to get a perfect 800 on the SAT math section, written by a perfect-scorer.
No comments:
Post a Comment